Home > Topdrawer > Fractions > Activities > Comparing non-unit fractions
Comparing non-unit fractions
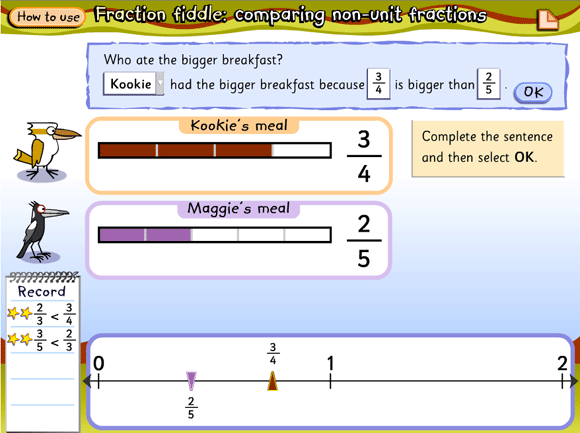
Display the learning object L2803 Fraction fiddle: comparing non-unit fractions to the class on an interactive whiteboard (or projector and screen).
Screen grab from L2803 Fraction fiddle: comparing non-unit fractions.
Source: © Education Services Australia Ltd, 2011
- Work through a task together, pausing for students to make predictions.
- Which fraction do you think is smaller/larger?
- Why do you think that?
- Encourage students to notice the relationships between the numerator/denominator and the construction of the fraction bar.
- What happens to the bar as the denominator gets larger?
- What happens to the bar as the numerator gets larger?
- Encourage students to notice the position of the fractions on the number line.
- Look where \(\frac{3}{4}\) is located. Where would \(\frac{1}{4}\) be? What about \(\frac{1}{2}\)?
- Is \(\frac{2}{5}\) going to be closer to 1 than \(\frac{3}{4}\) or further away? Why do you think so?
Students can work in pairs at computers to complete a set of tasks. The printed record of their findings can be the basis of further discussions about strategies for solving similar tasks without the learning object.
- What can you do to help you work out which fraction is larger or smaller?
- Why can’t you decide just by looking at the denominators?
