Home > Topdrawer > Fractions > Activities > Grids and jumps
Grids and jumps
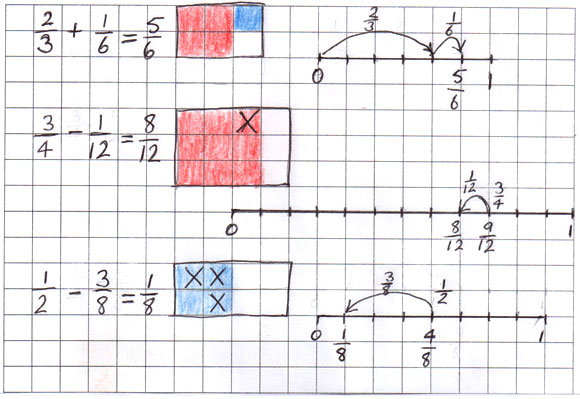
Work through at least one example with the class (e.g. \(\frac{1}{6}\) + \(\frac{2}{3}\)) demonstrating the construction of an appropriate labelled grid diagram, a number line showing the jumps and an equation.
Focus on identifying the multiplicative relationship between the denominators. For example, if working with sixths and thirds, recognising that 2 \(\times\) 3 = 6 leads to constructing a 2 \(\times\) 3 grid to show both the sixths and the thirds.
Although using a 6 \(\times\) 3 grid will also lead to a correct solution, encourage the students to find the smallest factor pair (making the smallest grid).
Select and prepare several sets of the task cards.
- Pairs of students select a task card and work together to present a solution.
- Grid paper can be used to support the construction of diagrams and number lines.
- Pairs who have worked on the same task cards can compare solutions and explain any differences.
As a class, discuss strategies for solving the addition or subtraction tasks without supporting diagrams.
In small groups students discuss the following scenario.
Someone in the class gave this solution: \(\frac{3}{10}\) – \(\frac{1}{5}\) = \(\frac{2}{5}\).
How would you explain why the answer is incorrect? Share some explanations as a class.