Home > Topdrawer > Fractions > Activities > Number lines
Number lines
This activity uses eighths, but can be adjusted to use other fractions and diagrams. Examples of same denominator problems are available.
Together the class constructs a number line from 0 to 2, labelled with eighths. The line should be labelled with both improper fractions and mixed numbers.
Present a contextual problem.
The family bought some pizzas. I ate \(\frac{4}{8}\) of the pepperoni pizza and \(\frac{3}{8}\) of the ham and pineapple pizza. How much pizza did I eat?
- Ask students to predict whether the answer will be less than, equal to, or more than one, and explain their reasons.
- Use questioning to scaffold the modelling of the problem with circles partitioned into eighths to represent the pizzas.
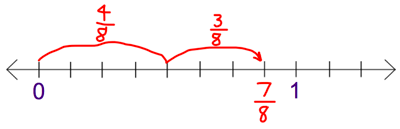
- On the number line, mark the two jumps to \(\frac{7}{8}\). Display the equation \(\frac{4}{8}\) + \(\frac{3}{8}\) = \(\frac{7}{8}\).
Student work sample showing addition of fractions with related denominators.
Extend the problem.
Later I ate \(\frac{2}{8}\) of the vegetarian pizza. How much pizza had I eaten altogether?
- Ask students to predict the answer, then model with the circle diagrams and the extra jump on the number line. Discuss the equivalence of \(\frac{9}{8}\) and 1\(\frac{1}{8}\).
- Display the equation: \(\frac{4}{8}\) + \(\frac{3}{8}\) + \(\frac{2}{8}\) = \(\frac{9}{8}\) = 1\(\frac{1}{8}\).
- In pairs, students work on a few similar problems, recording their diagrams and solutions. You might find the recording template useful.
As a class, review the solutions and discuss strategies for completing similar additions without the use of diagrams or number lines.
A similar approach can be used with subtraction problems.
